Nano Drive for Biomedical Science and Research

Introduction

Nano piezo drive on the reverse piezo effect is applied for conversion energy in the nano systems for biomedical science and research [1-8]. on the piezoelectric or electrostriction effects are used for nano movements in the precision equipment. The energy conversion in the structural scheme of the nano drive is visibility and logical in the difference from the conversion from the Cady’s and Mason’s schemes [7-9]. The structural model and scheme of nano drive are constructed from it matrix equations and differential equation for the drive [8-16].

Nano drives are used for atomic force microscopy, nano manipulators, nanotechnology, biotechnology, astronomy, space research, metrology, laser resonator [16-25].

Model of Nano Drive

Let us consider the deformation of the nano drives for biomedical science and research. Two matrix equations [8,11-16] for the nano piezo drive have the form

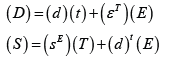
Where (D), (S), (T), (E) are matrices for electric induction, relative deformation, mechanical field and electric field stresses, t is matrix transpose operator.

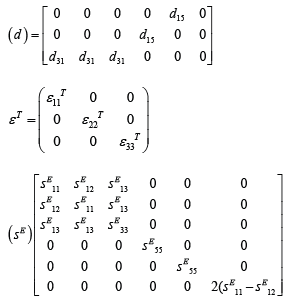
Matrices for the piezo modules, the dielectric constants and the elastic compliances for the nano piezo drive from PZT have the form

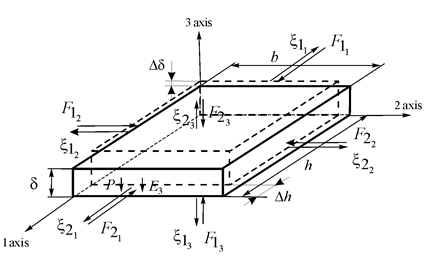
The equation of the reverse piezo effect [8, 11-16] for nano piezo drive on Figure 1 has the form

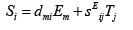
where m, i, j are axises (Figure 1).

Figure 1: Nano drive for nano biomedical science and research.

Differential equation for nano drive in nano biomedical science and research has the form [11-48].

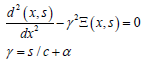
where x is coordinate, is operator, are coefficients, is speed of sound.

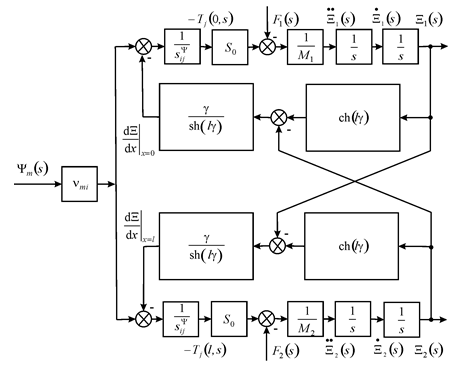
The equations structural model for nano drive has the form

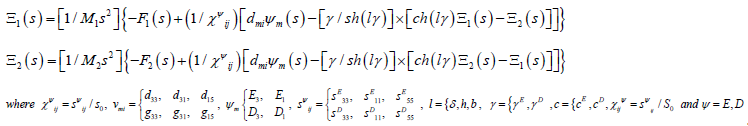
is the control parameter on Figure 2, is the length nano drive, are the masses.

Figure 2: Structural scheme nano drive for nano biomedical science and research.

Structural scheme nano drive on Figure 2 is used for calculation its deformations in nano systems for biomedical science and research instead Cady’s and Mason’s schemes (Figure 2).

Deformations of Nano Drive

Therefore, for nano drive its matrix equation deformations has the form

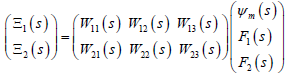
The steady state for its deformations have the form

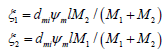
The steady state for its deformations for the transverse piezo drive have the form

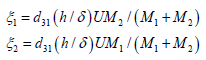
For the nano piezo drive from PZT = 0.25 nm/V, =10, = 0.5 kg, = 2 kg and = 50 V its steady-state deformations are determined in the form = 100 nm, = 25 nm, = 125 nm. Theoretical and practical deformations for nano piezo drive are coincidences with an error of 10%.

Conclusions

The structural model for nano drive is determined from matrix equations and differential equation for the drive. The equations structural model for nano drive are obtained for nano systems in biomedical science and research.

References

• Schultz J, Ueda J, Asada H (2017) Cellular Actuators. Butterworth-Heinemann Publisher pp. 382.
• Afonin SM (2006) Absolute stability conditions for a system controlling the deformation of an electro magnetoelastic transducer. Doklady Mathematics 74(3): 943-948.
• Uchino K (1997) Piezoelectric actuator and ultrasonic motors. Kluwer Academic Publisher Pp. 350
• Afonin SM (2005) Generalized parametric structural model of a compound electro magnetoelastic transducer. Doklady Physics 50(2): 77-82.
• Afonin SM (2008) Structural parametric model of a piezoelectric nano displacement transducer. Doklady Physics 53(3): 137-143.
• Afonin SM (2006) Solution of the wave equation for the control of an electro magnetoelastic transducer. Doklady Mathematics 73(2): 307-313.
• Cady WG (1946) Piezoelectricity: An introduction to the theory and applications of electromechancial phenomena in crystals. Mc Graw-Hill Book Company pp. 806.
• Mason W (1964) Physical Acoustics: Principles and Methods. Academic Press pp. 515
• Y Yang, L Tang (2009) Equivalent circuit modeling of piezoelectric energy harvesters. Journal of Intelligent Material Systems and Structures 20(18): 2223-2235.
• Zwillinger D (1989) Handbook of Differential Equations. Academic Press pp.673
• Afonin SM (2006) A generalized structural-parametric model of an electro magneto elastic converter for nano- and micrometric movement control systems: III. Transformation parametric structural circuits of an electro magneto elastic converter for nano- and micrometric movement control systems. Journal of Computer and Systems Sciences International 45(2): 317-325.
• Afonin SM (2016) Decision wave equation and block diagram of electro magneto elastic actuator nano- and microdisplacement for communications systems. International Journal of Information and Communication Sciences 1(2): 22-29.
• Afonin SM (2015) Structural-parametric model and transfer functions of electroelastic actuator for nano- and microdisplacement. Journal of Applied Mechanical 225-242.
• Afonin SM (2017) A structural-parametric model of electro elastic actuator for nano- and micro displacement of mechatronic system. Advances in Nanotechnology pp. 259-284.
• Afonin SM (2018) Electro magnetoelastic nano- and micro actuators for mechatronic systems. Russian Engineering Research 38(12): 938-944.
• Afonin SM (2012) Nano- and micro-scale piezomotors. Russian Engineering Research 32(7-8): 519-522.
• Afonin SM (2007) Elastic compliances and mechanical and adjusting characteristics of composite piezoelectric transducers. Mechanics of Solids 42(1): 43-49.
• Afonin SM (2014) Stability of strain control systems of nano-and microdisplacement piezotransducers. Mechanics of Solids 49(2): 196-207.
• Afonin SM (2017) Structural-parametric model electromagnetoelastic actuator nanodisplacement for mechatronics. International Journal of Physics 5(1): 9-15.
• Afonin SM (2019) Structural-parametric model multilayer electromagnetoelastic actuator for nanomechatronics. International Journal of Physics 7(2): 50-57.
• Afonin SM (2021) Calculation deformation of an engine for nano biomedical research. International Journal of Biomed Research 1(5): 1-4.
• Afonin SM (2021) Precision engine for nanobiomedical research. Biomedical Research and Clinical Reviews 3(4): 1-5.
• Afonin SM (2016) Solution wave equation and parametric structural schematic diagrams of electromagnetoelastic actuators nano- and microdisplacement. International Journal of Mathematical Analysis and Applications 3(4): 31-38.
• Afonin SM (2018) Structural-parametric model of electromagnetoelastic actuator for nanomechanics. Actuators 7(1): 1-9.
• Afonin SM (2019) Structural-parametric model and diagram of a multilayer electromagnetoelastic actuator for nanomechanics. Actuators 8(3): 1-14.
• Afonin SM (2016) Structural-parametric models and transfer functions of electro magneto elastic actuators nano- and microdisplacement for mechatronic systems. International Journal of Theoretical and Applied Mathematics 2(2): 52-59.
• Afonin SM (2010) Design static and dynamic characteristics of a piezoelectric nanomicrotransducers. Mechanics of Solids 45(1): 123-132.
• Afonin SM (2018) Electromagnetoelastic Actuator for Nanomechanics. Global Journal of Research in Engineering: A Mechanical and Mechanics Engineering 18(2): 19-23.
• Afonin SM (2018) Multilayer electromagnetoelastic actuator for robotics systems of nanotechnology. EEE Conference EIConRus, pp. 1698-1701.
• Afonin SM (2018) A block diagram of electromagnetoelastic actuator nanodisplacement for communications systems. Transactions on Networks and Communications 6(3): 1-9.
• Afonin SM (2019) Decision matrix equation and block diagram of multilayer electromagnetoelastic actuator micro and nanodisplacement for communications systems. Transactions on Networks and Communications 7(3): 11-21.
• Afonin SM (2020) Condition absolute stability control system of electromagnetoelastic actuator for communication equipment. Transactions on Networks and Communications 8(1): 8-15.
• Afonin SM (2020) A Block diagram of electromagnetoelastic actuator for control systems in nanoscience and nanotechnology, Transactions on Machine Learning and Artificial Intelligence 8(4): 23-33.
• Afonin SM (2020) Optimal control of a multilayer electroelastic engine with a longitudinal piezoeffect for nanomechatronics systems. Applied System Innovation 3(4): 1-7.
• Afonin SM (2021) Coded сontrol of a sectional electroelastic engine for nanomechatronics systems. Applied System Innovation 4(3): 1-11.
• Afonin SM (2020) Structural scheme actuator for nano research. COJ Reviews and Research 2(5): 1-3.
• Afonin SM (2018) Structural-parametric model electroelastic actuator nano- and microdisplacement of mechatronics systems for nanotechnology and ecology research. MOJ Ecology and Environmental Sciences 3(5): 306‒309.
• Afonin SM (2018) Electromagnetoelastic actuator for large telescopes. Aeronautics and Aerospace Open Access Journal 2(5): 270-272.
• Afonin SM (2019) Condition absolute stability of control system with electro elastic actuator for nano bioengineering and microsurgery. Surgery & Case Studies Open Access Journal 3(3): 307-309.
• Afonin SM (2019) Piezo actuators for nanomedicine research. MOJ Applied Bionics and Biomechanics 3(2): 56-57.
• Afonin SM (2019) Frequency criterion absolute stability of electromagnetoelastic system for nano and micro displacement in biomechanics. MOJ Applied Bionics and Biomechanics 3(6): 137-140.
• Afonin SM (2020) Multilayer piezo engine for nanomedicine research. MOJ Applied Bionics and Biomechanics 4(2): 30-31.
• Afonin SM (2020) Multilayer engine for microsurgery and nano biomedicine. Surgery & Case Studies Open Access Journal 4(4): 423-425.
• Afonin SM (2021) Structural diagram of actuator for nanobiotechnology. Open Access Journal of Biogeneric Science and Research 7(4): 1-6.
• Afonin SM (2021) Rigidity of a multilayer piezoelectric actuator for the nano and micro range. Russian Engineering Research 41(4): 285-288.
• Afonin SM (2021) Calculation of the deformation of an electromagnetoelastic actuator for composite telescope and astrophysics equipment. Physics & Astronomy International Journal 5(2): 55-58.
• Nalwa HS, editor (2004) Encyclopedia of Nanoscience and Nanotechnology. Los Angeles: American Scientific Publishers. 10 Volumes.
• Bhushan B, editor (2004) Springer Handbook of Nanotechnology. New York: Springer p. 1222.